A. ARTÍCULOS

 

Effective Hamiltonian for a tight-binding square lattice and its relation
to a two-mesh LC circuit with discrete charge

 

Hamiltoniano efectivo de una red cuadrada de enlace fuerte y su relación con un
circuito LC de dos mallas con carga discreta

 

 

Evaristo Mamani C., Marcelo Calcina-Nogales , Diego Sanjinés Castedo^†
Instituto de Investigaciones Físicas, Universidad Mayor de San Andrés
Campus Universitario, c. 27 Cota-Cota, Casilla de Correos 8635
La Paz - Bolivia
^†diegosanjinescastedo@gmail.com
Recibido 2 de septiembre de 2021; aceptado 18 de noviembre de 2021

 

 

---

Resumen

En este trabajo consideramos una función hamiltoniana de enlace fuerte extendida a primeros y segundos vecinos para una partícula cargada que se transporta por saltos (hopping) en una red cuadrada en presencia de un campo estático arbitrario y un campo uniforme rápidamente oscilante con frecuencia ω. La aplicación del método semiclásico y el método de Kapitza de promediación temporal hasta O(ω^-2) conduce a una función hamiltoniana efectiva (independiente del tiempo) con elementos de salto que dependen de los parámetros de los campos externos. Controlando dichos parámetros podemos manipular las interacciones de tal forma de emular un sistema físico diferente, en este caso, un circuito LC de dos mallas con carga discreta.

Descriptores: Método de enlace fuerte - modelo semiclásico - sistemas mesoscópicos.

---

Código(s) PACS: 31.15.aq, 03.65.Sq , 73.23.-b

---

Abstract

We consider an extended tight-binding Hamiltonian function comprising nearest and next-to-nearest neighbor interactions for a charged particle hopping in a square lattice in the presence of a static arbitrary field and a rapidly oscillating uniform field with frequency ω. The application of the semiclassical method and the Kapitza's method for time-averaging up to O(ω^-2) yields an effective (time independent) Hamiltonian function with long range hopping elements that depend on the parameters of the external fields. By controlling these parameters we can engineer the interactions in such a way as to emulate a different physical system, namely, a two-mesh LC circuit with discrete charge.

Subject headings: Tight-binding method - semiclassical model - mesoscopic systems.

---

 

 

1. Introduction

The study of effective Hamiltonians in solid state physics, derived with time-averaging procedures (originally due to P. L. Kapitza for the study of the inverted pendulum Kapitza, [1965,Landau, [1985] applied to tight-binding lattices, has recently acquired interest given the feasibility of managing (engineering) the hopping elements when the lattice is subject to external and rapidly oscillating driving fields. Some of the effects relevant for transport phenomena include, for example: dynamic localization, coherent control of tunneling, metal-insulator transitions, atomic motion in atom traps, effective next-to-nearest neighbor interactions and effective Bloch oscillation Dunlap, [1986,Rahav, [2003,Bandyopadhyay, [2008,Itin, [2014,Itin, [2015,Mamani, [2017]. The idea of using an extended tight-binding Hamiltonian with a kinetic energy of the form can be traced back to the work of Dunlap and Kenkre, Dunlap, [1986] where their results concerning dynamic localization are extended to long-range interactions in a 1D lattice with hopping elements A_n and lattice constant a. The case where all the interactions are considered led to the new concept of exact dynamic localization in the presence of an AC electric field Dignam, [2002]. Such a concept of "band engineering" with long-range interactions has been also investigated (theoretically and experimentally¹ in optical lattices; Longhi, [2010,Madison et al., [1998] reported the first observation of dynamical suppression of the band due to an external AC field in an optical lattice whereby the bandwidth shrinks to zero and the Bloch states become localized when the field amplitude meets a condition that was also derived in Mamani et al. Mamani, [2017] using the semiclassical method.² More recent investigations in layered graphene systems show that the effects derived from an extended tight-binding Hamiltonian can be considered as improvements to the usual nearest neighbor model results Reich, [2002,Kundu, [2011,Wright, [2009,Kadirko, [2013].

In this work we use an extended tight-binding Hamiltonian where the long-range interactions correspond to a bidimensional system, namely, an square lattice; then, we set about establishing a formal equivalence between that lattice and a two-mesh LC circuit with discrete charge by means of deriving and comparing their corresponding effective Hamiltonians (the case of a 1D lattice and its relation to the single-mesh LC circuit with discrete charge has been already studied by Mamani et al. Mamani, [2018]. We show that such an equivalence is possible by managing the parameters of the driving oscillating field (with frequency ω) acting upon the square lattice such that the nearest neighbor interactions become effectively suppressed leaving the remanent next-to-nearest neighbor (2nd neighbor) interactions as the dominant ones at order ω⁰ plus the 3rd neighbor interactions at order ω^-2. The theoretical framework for the study of quantum circuits with discrete charge is referred to Chen and Li, [1996] wherein the fundamental commutator is defined: nq_e are the discrete eigenvalues of the electric charge operator (n is an integer and q_e is the elementary electronic charge), the flux operator is the conjugate of and the substitution takes into account the discrete nature of the electric charge Flores, [2005,Calcina-Nogales, [2013,Flores, [2002]. The physical phenomena associated to these kind of systems have been reported: persistent currents, Bloch oscillations in quantum circuits, Coulomb blockade, current magnification, voltage and current engineering Chen and Li, [1996,Flores, [2005,Flores, [2002,Chen, [2005,Calcina-Nogales, [2020], etc. These cases demonstrate the feasibility of modeling the physics of mesoscopic devices within the conceptual framework referred to above.

Although the one-dimensional and the square tight-binding lattices do not exist as such (to our knowledge), it seems that the reported discrete-charge mesoscopic systems would be soon technicaly feasible, thus, permitting the posibility of testing the predictions deduced from comparing both systems. In this sense, the semiclassical method together with the time-averaging techniques provide an easier and more straightforward way of deriving an effective Hamiltonian, as we could in fact verify in the derivation of the quantum effective Hamiltonian of the two-mesh LC circuit Calcina-Nogales, [2020]. Besides, the semiclassical method also provides a bifurcation condition already studied in the one-dimensional lattice Mamani, [2018] and suggested in the two-dimensional case (in this work) that could be useful for predicting the transition between different electronic dynamical regimes.

The organization of our work is the following: in Section 2 we derive the effective Hamiltonian for a square lattice using the time-averaging technique and extending the procedures we have already used for the one-dimensional lattice Mamani, [2017]; in Section 3 we apply the results of the previous section to the managing of the effective Hamiltonian hopping elements so as to emulate case of the two-mesh LC circuit with discrete charge; finally, in Section 4 we present the most important concluding remarks and point out some directions for future research.

 

2. Derivation of the effective Hamiltonian for the square lattice

Consider the extended tight-binding Hamiltonian function with nearest-neighbor and next-to-nearest neighbor interactions for an independent test particle with charge q_e (i.e., we do not consider any possible interaction with like particles whatsoever neither the particle affects the distribution of the external electric fields acting upon it) which hops in a square lattice with cells of side a under the action of external electric fields,

The tight-binding band in the Hamiltonian (1) has the standard form where k=(k_x,k_y) and R_m,n=a(m,n); the hopping elements are A = γ_0,±1= γ_±1,0 for the nearest neighbors and C = for the next-to-nearest neighbors. In the following derivation, and in order to deal with a more compact notation, we take unitary numerical values for the lattice constant a and for the physical constants q_e, ħ. V(r) is an external arbitrary static potencial energy at r=(x,y) and f(ωt)=(f_x,f_y) is the rapidly oscillating external driving field with frequency ω >> 1/T, where T is the particle's characteristic period of oscillation in the absence of the driving field. Without loss of generality and for the sake of simplicity, we will suppose that the external electric field f(ωt) is an even function of t.

Although the quantum Hamiltonian corresponding to with V(r)=0 has the spatial divergent potential r ·f(ωt), the problem of finding its eigenfunctions has been already widely treated in terms of the vector potential representation Houston, [1940,Krieger, [1986,Kittel, [1987,Rossi, [1997] whereby the electric field is f(ωt)= ∂g/∂t, such that the momentum operator is shifted as p → p+ g within the kinetic energy operator. As a consequence, the "acceleration theorem" ∂k/∂t=f(ωt) is derived strictly quantum-mechanically in agreement with the semiclassical approach considered in our work.³ For the case of V(r) ≠ 0 in , the gauge substitution g → g+ ∇V(r)t is made and the resulting acceleration theorem becomes ∂k/∂t=f(ωt)-∇V(r).

We use now the Hamilton's equations which yield the time derivatives of the position and the momentum:

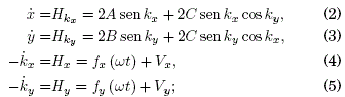
we set the notation V_x, V_y, V_xx, V_yy, V_xy=V_yx and H_kx, H_ky, H_x, H_y for the derivatives of V(r) and H(r,k;t) respectively; this notation will apply only to the Hamiltonian and potential energy functions hereafter. It will be convenient also to use the generic symbol z for either of the coordinates x,y where a simplified expression could be written. Let us now apply the canonical transformations between the momenta: (z,k_z)→(z,k’_z) given by k’_z ≡ k_z+g_z, whereby the "displaced momentum" k’_z is defined along with the time integral of the external field, ; this is the realization of the vector potential representation that yielded k → k+ g as projected onto the square lattice and which was already referred to. The substitution of these transformations in (2)-(5) yields:

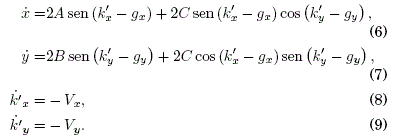
Due to the action of the combined static and oscillating fields, -∇V(x,y) and f(ωt), the particle will move with small oscillations around a slow varying trajectory. Thus, we introduce the "slow" Z(t), K_z(t) coordinates and the "fast" ξ_z(τ), η_z(τ) coordinates in the direct and reciprocal spaces respectively; the fast coordinates are considered perturbations of the slow coordinates:

where τ ≡ ωt is such that the time-average of ξ_z(τ), η_z(τ), vanish in the time interval with period T=2π/ω while Z(t), K_z(t), remain almost constant in the same interval, i.e., ⟨ ξ_z ⟩ = ⟨ η_z ⟩ =0, and ⟨ Z ⟩ =Z(t), ⟨ K_z ⟩ =K_z(t). We have used the definition of the time-average as

The set of transformations

is canonical since the structure of Hamilton's equations is preserved Landau, [1985]:

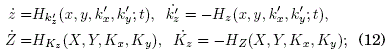
this is so as a consequence of the invariance of the Poisson brackets: [k_z,z]=[k’_z,z]=[K_z,Z]=1 which yields H(x,y,k’_x,k’_y;t)=H(x,y,k_x,k_y;t)+∂F(x,y,k’_x,k’_y;t)/∂t for some generating function F(x,y,k’_x,k’_y;t) that can be readily calculated, and the time-averaging which gets us from H(x,y,k’_x,k’_y;t) to H(X,Y,K_x,K_y). The resulting shift of the time dependence from the r ·f(τ) term in H(x,y,k_x,k_y;t) into the arguments of the kinetic energy operators in H(x,y,k’_x,k’_y;t) is not only a convenient transformation (in order to get time-averages efficiently) but it is also a necessary one to render k=(k_x,k_y) as a "good quantum number" Kittel, [1987]. Now, H(X,Y,K_x,K_y) ≡ H^eff is the form of the effective Hamiltonian that, as a result of the Hamilton's equations, represents a constant of motion and whose explicit construction will be possible giving the resulting Eq.(7) at the end of this section. In this work we will restrict the potential energy to the quadratic form of the position coordinates V(x,y)=c₁ x²+c₂ y²+c₃ xy since it is this kind of function that yields the correct physical interpretation for H^eff as a tight-binding Hamiltonian (the physical realization of such V(x,y) onto the square lattice plane would be achieved -in principle- by placing the plane inside a dielectric cylinder shell with an specific surface charge distribution); otherwise, if V(x,y) had the form of a higher degree polynomial function, H^eff could still be a valid Hamiltonian but not with the form of a tight-binding one Mamani, [2017].

By replacing the time-derivatives of the terms in (12) into the system (6)-(9) we obtain the system (B1)-(B4) in Appendix B wherein we applied the time-average techniques that yield the system for the effective dynamical coordinates:

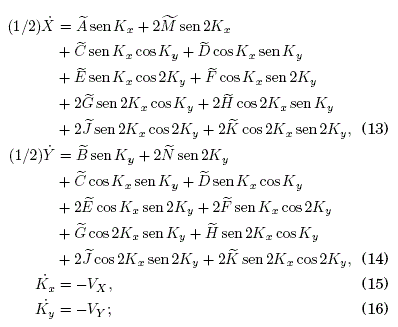
the list of the "tilde" symbols used in (13) and (14) is defined in the Appendix A. We now set up about constructing the effective Hamiltonian H^eff ≡ H(X,Y,K_x,K_y) from the system -. The Hamilton equations in the space of the effective coordinates Z,  K_z must be satisfied:

the expression for in (17) is substituted from (13), (14) and combined together with (15), (16) to give

where Ω is a constant term independent of the dynamical coordinates Z,  K_z. It is now clear that the "tilde" symbols referred to in (13) and (14) constitute the effective hopping elements characteristic of the effective tight-binding Hamiltonian H^eff in (18).

As a first crosscheck calculation of (18) we can test it for the case of the null electric field f=0 and a linear static potential V(X,Y)=αX+βY (dropping the constant term Ω):

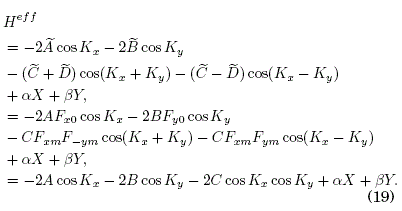
By using Hamilton's equations in (17) for Z=X,Y, we obtain

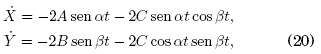
which describe, as expected, a 2D Bloch oscillation with period T=2pπ/α  (p=min(n,m)) for the rational quotient α/β = n/m.

 

3.  Interactions engineering and relation to an LC circuit

With the explicit form of the effective Hamiltonian H^eff in (18) in terms of the Fourier components f_zn of the electric field via (B31), we may ask now which components will yield specific values of the effective hopping elements in H^eff that determine thus the transport properties of the particle in a square lattice when acted upon by the external fields. This is the "interactions engineering" scheme considered in this paper, particularly, as an extension of the one-dimensional case investigated by Mamani et al. Mamani, [2017]. For the purpose of illustrating such interactions engineering, we choose as a case study the emulation of an LC circuit with discrete charge that has an effective Hamiltonian of the form (as we will see later in this section)

where the static potential is the quadratic form V(X,Y) ∞ X²+Y²+(X-Y)² such that its second derivatives are V_XX=V_YY=-2 V_XY. In this case, and for the square lattice with A=B, the effective hopping elements become (Appendix A):

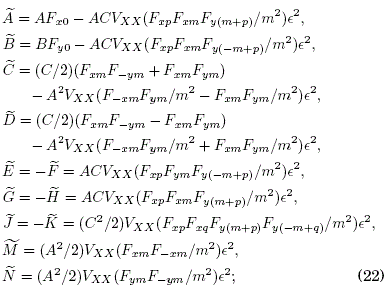
sums are performed on the terms with the repeated indices m,  p,  q and the variable m. Since our perturbative calculations were performed consistently up to second order in ∈ ≡  1/ω, the effective hopping elements in (22) can be expressed as combinations of the zeroth (dominant) and 2nd order terms: .

Now, the F_zm terms in (22) depend on the specific oscillating electric field f=(f_x,f_y) through its Fourier components. For the purpose sought in this work, we find that those components should be

Then, the expression for F_zm in (B31) readily becomes

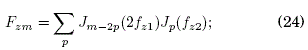
notice the property F_(-z)m= F_z(-m) ≡ F_-zm. In order to solve the infinite sum in (24) and verify its convergence, we use the integral representation for J_n(z):

Replacing this J_n(z) in F_zm and using we obtain

Now, we set f_x2= f_y1= 0 in (23) as the condition to find the required form of H_LC ( in this case) with the parameters f_x1,  f_y2 such that:

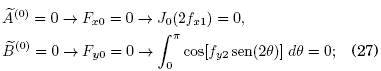
then, we chose from the set of solutions {f_x1,f_y2} of those which satisfy the condition that should be negligible as compared to and . One way of doing this is to calculate the euclidian norm

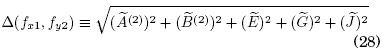
such that attains a minimum value for negative values of Thus, with this condition and those of (27) fulfilled, the effective Hamiltonian in (18) becomes

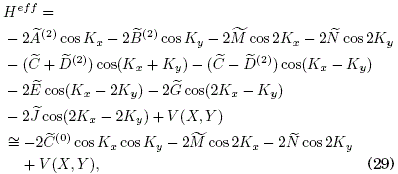
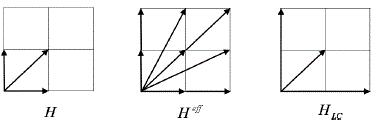
thus attaining the required approximation . Fig. 1 shows an schematic representation of the interactions in the square lattice (from left to right): the time-dependent Hamiltonian H in (1) is transformed into the effective Hamiltonian in H^eff (18) (with all the reparametrized and induced interactions) by the action of the external oscillating and static fields and, finaly, by choosing specific values of the those fields ("interactions engineering"), the Hamiltonian H_LC in (29) is obtained.

Figure 1: Representations of the interactions in the square lattice (from left to right): the time-dependent
Hamiltonian H in , the effective Hamiltonian H^eff in , the Hamiltonian H_LC in obtained by choosing specific
values of the external fields (interactions engineering).

The numerical results found from (27) and (28) within the interval 0 < f_x1,f_y2 < 100 (with physical units restored) are

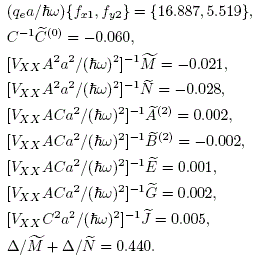
We expect that V_XX A²a²/(ħ ω)² has an upper bound in order to have consistent results, i.e., dominant with respect to and . This is of course the case when but, as we will see at the end of this section, ω must also have an upper bound if an analogy between the square lattice and the LC circuit is to be achieved. We think that values of the physical parameters can be found that meet reasonably such a requirement.

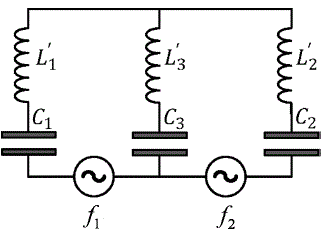
Figure 2: Two-mesh LC circuit.

We now set up about describing the LC circuit depicted in Fig. 2. Following the construction of the quantum Hamiltonian operator for a two-ring system Flores, [2002], we can construct the Hamiltonian function of a two-mesh LC circuit with discrete charge q_e as

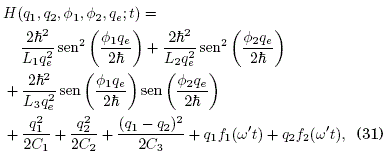
which follows from the replacement of the magnetic flux as in the corresponding continuous-charge Hamiltonian function

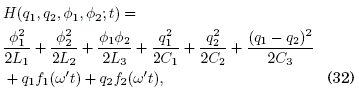
where we have defined: 1/L₁ ≡ (L₂’+L₃’)/Υ, 1/L₂ ≡ (L₁’+L₃’)/Υ and 1/L₃ ≡ 2 L₃’/Υ, with Υ ≡ (1/2)∑_{i ≠ j} L_i’L_j’; as can be readily checked the flux φ_i remains invariant in the limit q_e → 0 yielding, as required, H(q₁,q₂,φ₁,φ₂,q_e;t) → H(q₁,q₂,φ₁,φ₂;t). In this case, the term φ₁ φ₂/(2 L₃) in (32) is derived from the energy term (1/2)L’₃(·q₁-·q₂)² according to the Kirchhoff's laws. The mutual inductance terms corresponding to this circuit are φ₁ φ₂/M₁₂, φ₁ φ₃/M₁₃ and φ₂ φ₃/M₂₃ but we have not considered them in (32) since we did not specify the form of the couplings among L’₁, L’₂ and L’₃. In fact, the "mesoscopic" character of this circuit lies upon the discrete nature and small quantity (about 10) of the elementary electric charges being allocated in the capacitors and not necessarily upon the small size of the circuit which would imply couplings among the inductors.⁴

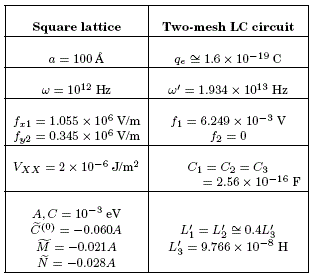
Table 1: Numerical values of the physical parameters. The values of a, ω, A, C were chosen so as to
resemble typical values in a GaAs-type semiconductor superlattice; f₂=0 was suggested so as to
facilitate the calculations. The other values result from comparing the terms and arguments of the
effective Hamiltonians and , and from the corresponding algebraic and numerical manipulations.

Following the standard quantum time-average techniques Rahav, [2003,Calcina-Nogales, [2020] and setting the canonical transformations φ₁=Φ₁+πħ/q_e and φ₂=Φ₂+πħ/q_e, we obtain from (32) the effective Hamiltonian function⁵

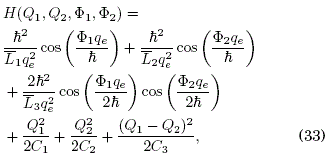
where, for the case of the AC source voltages f₁(ω’t)=2 f₁ cos(ω’t) and f₂(ω’t)=2 f₂ cos(2 ω’t), we obtain

By comparing the terms and arguments of H(Q₁,Q₂,Φ₁,Φ₂) in with those of H_LC(X,Y,K_x,K_y) in we can verify, as expected, that the required form of H_LC is attained for static potential energies V(Q₁,Q₂) and V(X,Y) whose second derivatives are related as V_{Q1 Q1}/V_XX=V_{Q2 Q2}/V_YY=V_{Q1 Q2}/V_XY=(a/q_e)² . Specifically, by choosing equal capacitances in , we should have that V_XX=2(q_e/a)²/C₁.

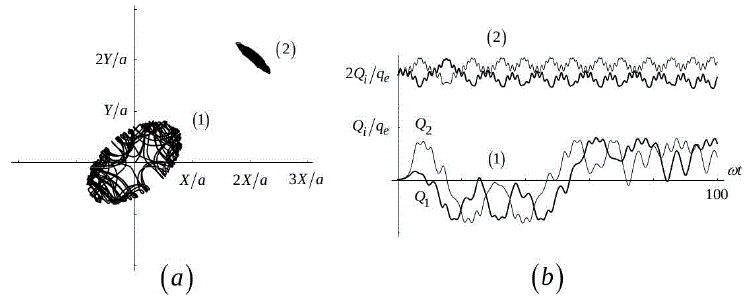
Figure 3: Cases of electronic motion in: (a) the effective position XY plane, and (b) the two-mesh LC circuit. In case
(a) the displacements are measured in units of the lattice constant a=100 A; in case (b) the capacitors' electric charges are
measured in units of the electron's charge q_e, the time is measured in units of the frequency inverse 1/ω = 10^-12 s.
Cases (1) and (2) in both (a) and (b) correspond to oscillations with zero and positive time-averages, respectively. Cases (1)
and (2) in (b) resemble very much the AC and DC regimes, respectively, deduced in the one-mesh LC circuit Mamani, [2018]
as a consequence of a bifurcation condition.

From the numerical values of f_x1, f_y2, , and found in , we may now express the equivalence among the coefficients of H(Q₁,Q₂,Φ₁,Φ₂) and H_LC(X,Y,K_x,K_y) in the compact notation that comprises the three equations

whence the the source voltage amplitudes f₁, f₂ and the inductances can be found. We may take L’₁=L’₂ for simplicity, thus, from in , we have that which is satisfied for an infinte set of solutions, for example, (q_e/ħω’) f₁=0.517 and (q_e/ħω’) f₂=0 (although f₂=0 was so chosen just to facilitate the calculations, such a value still deserves a further phyisical interpretation). For these values, and by setting , in the Hamiltonians (21), (33), respetively, we find

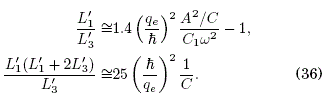
As we can see, the term (q_e/ħ)² (A²/C)/(C₁ ω²) has to have a lower bound to yield positive inductances; such a bound will be determined by the characteristics of the square lattice which, in this work, can be thought of as a 2D semiconductor superlattice of the GaAs type (and whose 1D version was where Bloch oscillations were first observed). Thus, we may take as typical values:

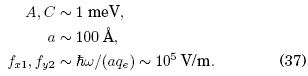
From the latter we have an estimation of ω 10¹² Hz. Since V_XX (Aa/ħ ω)²=(A q_e/ħ)²/(C₁ ω²) 10^-22 J should hold in (30), we have then the estimation of C₁ 10^-16 F. With these values, we have therefore from (36) that H. Finally, an estimation of the sources frequency ω’ can be made by assuming that a maximum of just few electrons is to be alloted in a (small) mesoscopic capacitor, which is other way for interpreting the meaning of "discrete charge" LC circuit. We may take then Q_1,max=10 q_e, which, together with V and ω’  10¹³ Hz. More precise values for the results of our numerical simulations and from comparing the terms and arguments of the effective Hamiltonians (33) and (21) are summarized in Table 1 .

We may now validate the consistency of the semiclassical model invoked in our work: the value of the frequency ω = 10¹² Hz corresponds to an external field wavelength λ  10^-3 m, while the lattice constant is a=100 A. The quantum wavepacket size can be taken from Mamani et al. Mamani, [2017], where the semiclassical method has proved to be consistent with the formal quantum approach for a wavepacket initial width Δx=10 a. We have therefore that λ >> Δx >> a, as required for the validity of the semiclassical method (see, for example Ashcroft and Mermin, [1976]), and which in turn implies that the intensity of the external electric field |f|   10⁶ V/m is low enough for a single-band tight-binding model Hamiltonian to hold.

By solving numerically the system of equations (17) for X(t), Y(t), Q₁(t), Q₂(t), with the values reported in Table 1, we may relate the electronic dynamics in both equivalent systems, the square lattice and the two-mesh LC circuit. Since we have managed the electric fields and the voltage sources in the former and latter systems, respectively, so as to have H_LC(X,Y,K_x,K_y) ≅ H_LC(Q₁,Q₂,Φ₁,Φ₂), then their common energies are 0.218 meV, corresponding to the initial values X(0)=Y(0)=0.02 a of the effective position coordinates. We observe in Fig. 3(a(1)) a predominately diagonal oscillating motion -although not a simple one- of the particle's position about the origin of the effective position XY plane of the square lattice, which corresponds also to a predominately symmetric distribution of oscillating charges in the two-mesh LC circuit, as seen in Fig. 3(b(1)), in accordance with the diagram for H_LC in Fig. 1, where the diagonal hopping element is the dominant one. Such a behavior, however, may change abruptly -as a function of the total energy, for example- giving rise to a DC regime or a supression of the mesh currents, as it does in the one-dimensional case of the one-mesh LC circuit Mamani, [2018], where we have showed that a bifurcation condition on the · Q-Q phase diagram can be associated to such an abrupt change. Figs. 3(a(2)) and 3(b(2)) show position and charge oscillations with positive time-averages corresponding to an energy of 2.707 meV, contrasting with those of Figs. 3(a(1)) and 3(b(1)). The extension of the one-dimensional bifurcation condition to the two-dimensional case of the two-mesh circuit and its relation to the particle's propagation in the square lattice is an interesting issue worth to be treated elsewhere.

 

4. Conclusions

We have established the formal equivalence between two physical systems by means of the interactions engineering scheme developed in this paper. Those systems are: (i) a square lattice wherein an tight-binding electron propagates under the combined action of an external high-frequency and homogeneous electric field and a quadratic static potential, and (ii) a two-mesh LC circuit with discrete charge. Such formal equivalence is attained by describing both systems by effective Hamiltonian functions having the same form and whose parameters can be numerically calculated when the Hamiltonian terms are correspondingly compared among them; for deriving such effective Hamiltonians we have used perturbative expansions up to ω^-2 when . Interestingly, we have found that the sought equivalence between the square lattice and the LC circuit is achieved when we choose specific values of the oscillating electric field acting upon the lattice so that the 1st neighbor interactions () are suppressed and the remaining 2nd neighbor interactions () and 3rd neighbor interactions (,, order ω^-2) become comparable (see Table 1).

We now suggest some interesting issues that could be worth exploring further: (a) In a previous work we have investigated the relation between a single-mesh LC circuit (with discrete charge) and a charged particle hopping in a one-dimensional lattice Mamani, [2018]; in the present work we have extended such an analogy to a two-mesh LC circuit and a square lattice. Thus, it seems natural to inquire whether the extension to a three-mesh LC circuit and a cubic lattice would be valid, mainly, because the one-dimensional and the square lattices do not exist as such (to our knowledge), but the three dimensional cubic lattice does exist (although not with the superlattice parameters specified in Table 1). (b) Another natural extension of our work would be considering a rectangular lattice where, for example, the sides have an incommensurate ratio of In fact, this latter case would be the best suited for simulating the dynamics of a hopping electron in graphene. (c) Finally, and motivated by the results in Fig. 3(a) for the square lattice, we may suggest that the "AC regime" would indicate a quantum regime where the electronic probability densities corresponding to neighbor lattice sites overlap, which indicates in turn a higher electric conductivity, as compared to the "DC regime". Thus, a higher and a lower conducting regimes could be separated by a semiclassical bifurcation condition.

 

Footnotes:

¹Many of the current theoretical models do not take into account some real physical effects (for simplicity), such as interband transitions, dispersion of the hopping particle by thermal collisions and phononic interactions, loss of crystal periodicity, etc., due which the particle's wavepacket usually decoheres rapidly and the observation -for example- of Bloch oscillations is restricted to a few complete periods (c.f.: Lyssenko et al., [1997,Madison et al., [1998]). In the absence of such effects, the wavepacket's width may oscillate and eventually be restored to its initial value, as shown, e. g., by Dignam and de Sterke in the exact dynamic localization effect Dignam, [2002].

²A concise and pedagogical justification of the semiclassical method for a general periodic potential can be found, for example, in Ch. 12 of Ashcroft and Mermin, [1976]

³In this sense, the semiclassical method provides exact results that coincide with the quantum ones, as we could verify in the above mentioned references and also in Dignam and de Sterke,Dignam, [2002] wherein a study of exact dynamical localization is carried on (we have work in progress in this direction).

⁴Interestingly, the phenomenon of current magnification referred to by Flores and Utreras Flores, [2002] for two coupled inductances would also occur in the two-mesh circuit with three inductances studied in our work; progress is being carried on by us in that direction.

⁵The resulting Hamiltonian operator corresponds to the Hamiltonian function obtained with the semiclassical scheme, as was already done in the case of the single-mesh LC circuit Mamani, [2018].

 

References

[Ashcroft and Mermin 1976]

    Ashcroft N. & Mermin N. D. 1976, Solid State Physics (Saunders College, Philadelphia).         [ Links ]

[Bandyopadhyay 2008]

    Bandyopadhyay M. & Dattagupta S. 2008, Pramana J. Phys. 70, 381.         [ Links ]

[Calcina-Nogales 2013]

    Calcina-Nogales M. 2003, Mod. Phys. Lett. B 27, 1350138.         [ Links ]

[Calcina-Nogales 2020]

    Calcina-Nogales M., Mamani E. & Sanjinés D. 2020, preprint.         [ Links ]

[Chen and Li 1996]

    Chen B. & Li Y. Q 1996, Phys. Rev. B 53, 4027.         [ Links ]

[Chen 2005]

    Chen B. 2005, Phys. Lett. A 335, 103.         [ Links ]

[Dignam 2002]

    Dignam M. M. & de Sterke C. M. 2002, Phys. Rev. Lett. 88, 4, 046806.         [ Links ]

[Dunlap 1986]

    Dunlap D. H. & Kenkre V. M. 1986, Phys. Rev. B 34, 3625-3633.         [ Links ]

[Flores 2002]

    Flores J. C. & Utreras-Díaz C. A. 2002, Phys. Rev. B 66, 153410.         [ Links ]

[Flores 2005]

    Flores J. C. & Lazo E. 2005, IEEE 4, 688.         [ Links ]

[Houston 1940]

    Houston W. V. 1940, Phys. Rev. 57, 184.         [ Links ]

[Itin 2014]

    Itin A. P. & Neishtadt A. 2014, Phys. Lett. A 378, 822.         [ Links ]

[Itin 2015]

    Itin A. P. & Katsnelson M. I. 2015, Phys. Rev. Lett. 115, 075301.         [ Links ]

[Kadirko 2013]

    Kadirko V., Ziegler K. & Kogan E. 2013, Graphene 2, 97.         [ Links ]

[Kapitza 1965]

    Kapitza P. L. 1965, in Collected Papers of P. L. Kapitza, edited by ter Haar D. (Pergamon Press, Oxford); Kapitza P. L. 1951, Zh. Eksp. Teor. Fiz. 21, 588.         [ Links ]

[Kittel 1987]

    Kittel C. 1987, Quantum Theory of Solids (John Wiley and Sons, New York).         [ Links ]

[Krieger 1986]

    Krieger J. B. & Iafrate G. J. 1986, Phys. Rev. B 33, 5494.         [ Links ]

[Kundu 2011]

    Kundu R. 2011, Mod. Phys. Lett. B 25, 163.         [ Links ]

[Landau 1985]

    Landau L. D. & Lifschitz E. M. 1985, Mecánica (Reverté, Barcelona).         [ Links ]

[Longhi 2010]

    Longhi S., Dreisow F., Heinrich M., Pertsch T., Tünnermann A., Nolte S. & Szameit A. 2010, Phys. Rev. A 82, 053813.         [ Links ]

[Lyssenko et al. 1997]

    Lyssenko V., Valusis G. & Löser F. 1997, Phys. Rev. Lett. 79, 301.         [ Links ]

[Madison et al. 1998]

    Madison K. W., Fischer M. C., Diener R. B., Niu Q. & Raizen M. G. 1998, Phys. Rev. Lett. 81, 23, 5093.         [ Links ]

[Mamani 2017]

    Mamani E., Calcina-Nogales M. & Sanjinés D. 2017, Int. J. Mod. Phys. B 31, 1750116.         [ Links ]

[Mamani 2018]

    Mamani E., Calcina-Nogales M. & Sanjinés D. 2018, Rev. Mex. Fis. 64, 456-463.         [ Links ]

[Rahav 2003]

    Rahav S., Gilary I. & Fishman S. 2003, Phys. Rev. A 68, 013820.         [ Links ]

[Reich 2002]

    Reich S., Maultzsch J., Thomsen C. & Ordejón P. 2002, Phys. Rev. B 66, 035412.         [ Links ]

[Rossi 1997]

    Rossi F. 1997, Bloch oscillations and Wannier-Stark localization in semiconductor superlattices (Theory of transport properties of semiconductor nanostructures, ed. E. Schoell, Chapman and Hall, London).         [ Links ]

[Wright 2009]

    Wright A. R., Liu F. & Zhang C. 2009, Nanotechnology 20, No. 40.         [ Links ]

 

Appendix

A Definitions of the effective hopping elements

The "tilde" symbols used in (13) and (14) that constitute the effective hopping elements of H^eff in (18) are defined as:

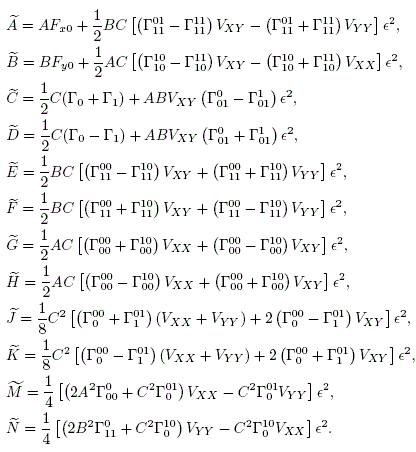
The definitions of the "Γ" symbols used above are:

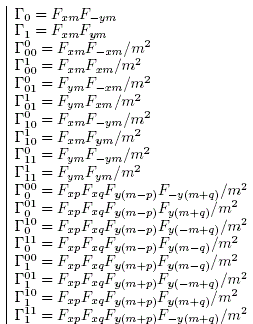
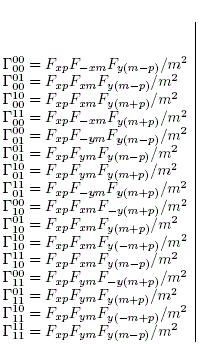
Sums are performed on the terms with the repeated indices m,  p,  q and the variable m.

B Time-average derivation of the effective dynamical coordinates

In this Appendix we apply the time-average techniques that yield the system for the effective dynamical coordinates (13)-(16). This is done by replacing the time-derivatives of the terms in (10) into the system (6)-(9) yielding:

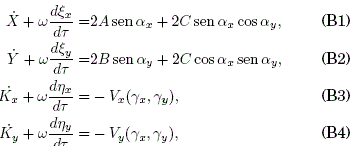
where we have defined α_z ≡ K_z+η_z-g_z, γ_z ≡ Z+ξ_z. We will use later α_z⁰ ≡ K_z+η_z0-g_z which results from evaluating α_z for ∈ = 0, and α_z⁰⁰ ≡ K_z-g_z which results from evaluating α_z⁰ for η_z0=0. The application of the time-average properties to the system (B1)-(B4) yields:

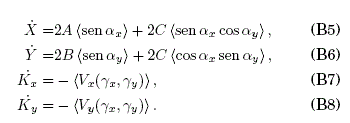
Combining (B5)-(B8) and (B1)-(B4) one obtains a system for the fast coordinates:

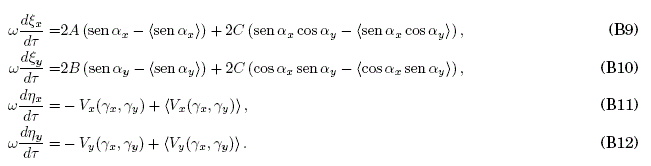
The solutions of (B9)-(B12) are to be substituted in (B5)-(B8) in order to have a system of differential equations for the slow coordinates Z,  K_z. To achieve that, and since the frequency ω is large, we expand the fast coordinates ξ_z,  η_z as power series of the small parameter ∈ ≡ t/τ = 1/ω up to the order of ∈² with coeficients ξ_zi,  η_zi. The substitution of these series in two of the trigonometric functions and in the derivatives V_x, V_y in (B5)-(B8) leads to

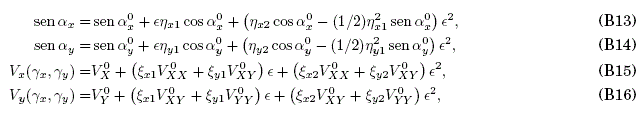
where γ_z0 ≡ Z+ξ_z0; the superscript "0" in the derivatives of the potential energy means that its argument is γ_z0 and the abscence of a superscript means that the argument is Z. Substituting (B13)-(B16) in (B9)-(B12), and comparing the terms in the same powers of ∈, one obtains:

(i) For ∈⁰,

(ii) For ∈¹,

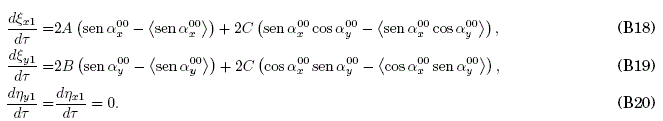
(iii) For ∈²,

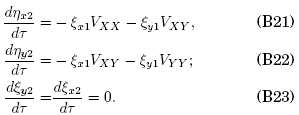
The convenient solutions for (B17), (B20) and (B23) are chosen as ξ_x0=η_x0=ξ_y0=η_y0=0, η_z1=0 and ξ_z2=0, respectively. The resulting right-hand side of the system (B5)-(B8) stands now as:

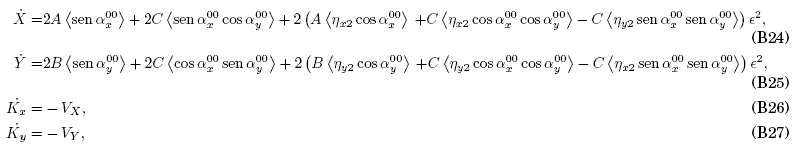
where α_z⁰⁰ depends on K_z and on the external fields, while η_z2 is to be found by solving (B18), (B19), (B21) and (B22). The result will be the sought system of differential equations for the slow coordinates Z,  K_z whose solution will permit us to deduce the effective Hamiltonian function H(X,Y,K_x,K_y).

Let us express the trigonometric functions in (B24), (B25) in complex form:

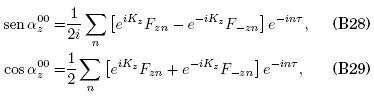
where we have used the following Fourier expansion given that g_z(τ) is a periodic real and odd function of t, corresponding to the real and even function f_z(τ):

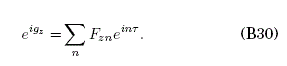
For the external field the coefficients F_zn are

Substituting now (B28), (B29) in (B18), (B19), and solving these along with (B21), (B22) for η_z2 we find:

where

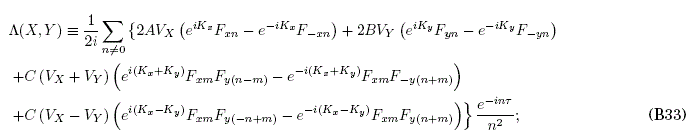
a sum in Λ is performed on the terms with the repeated index m. Substituting now (B32), (B28) and (B29) in (B24) and (B25), and performing the time-average operations with , we obtain the system for the effective dynamical coordinates X,  Y,  K_x,  K_y given in (B13)-(B16)